How To Draw An Incenter
How To Draw An Incenter - Learn how to construct the incenter of a triangle along with the incircle. Web in construction, we can find the incenter, by drawing the angle bisectors of the triangle. Click on next or run to begin. Inscribe a circle in a triangle. As can be seen in incenter of a triangle, the three angle bisectors of any triangle always pass through its incenter. Construct a perpendicular from the incenter to one side of the triangle to locate the exact radius. Find the coordinates of the incenter of a triangle whose vertices are given as a(20, 15), b(0, 0) and c. Use this information to help you. 1.3k views 3 years ago. This geometry video tutorial explains how to identify the location of the incenter, circumcenter, orthocenter and centroid of a triangle. This can be done by using a compass. Take any two sides of the triangle and find their midpoints. We bisect the two angles using the method described in bisecting an angle. Scroll down the page for more examples and solutions on the incenters of triangles. Web the incenter of a triangle is the point at which the three angle. Draw the perpendicular bisectors for the remaining sides of the triangle. Web in construction, we can find the incenter, by drawing the angle bisectors of the triangle. The steps to draw the angle bisectors are mentioned below: Locate the incenter by constructing the angle bisectors of at least two angles of the triangle. Web the following diagram shows the incenter. Construct a perpendicular from the incenter to one side of the triangle to locate the exact radius. Construct an angle bisector for one of the angles of the triangle. 1.3k views 3 years ago. Click on next or run to begin. Web now the above formula can be used: Web © 2024 google llc. The point where they all intersect is the incenter. Web now the above formula can be used: Try this drag any point a,b,c. Find the incenter of a triangle using a compass and straightedge at: Web now the above formula can be used: However, in coordinate geometry, we can use the formula to get the incenter. Inscribe a circle in a triangle. One of several centers the triangle can have, the incenter is the point where the angle bisectors intersect. Locate the incenter by constructing the angle bisectors of at least two angles of the. This construction is done by creating the angle bisectors. You can also drag the origin point at (0,0). The point where they all intersect is the incenter. Draw the perpendicular bisectors for the remaining sides of the triangle. Web this page shows how to construct (draw) the incenter of a triangle with compass and straightedge or ruler. 385 views 2 years ago relationships within triangles. Web learn how to construct the incenter of a triangle in this free math video tutorial by mario's math tutoring using a compass and straightedge. Use this information to help you. With three angle bisectors, you construct an incenter. Try this drag any point a,b,c. Locate the incenter by constructing the angle bisectors of at least two angles of the triangle. Inscribe a circle in a triangle. Web incenter (i) of a triangle = (ax 1 + bx 2 + cx 3 /a + b + c, ay 1 + by 2 + cy 3 /a + b + c), here vertices a = (0,. Construct an angle bisector for one of the angles of the triangle. Web to construct the incenter, first construct the three angle bisectors; Find the coordinates of the incenter of a triangle whose vertices are given as a(20, 15), b(0, 0) and c. This can be done by using a compass. Web in construction, we can find the incenter, by. This can be done by using a compass. Construct an angle bisector for another angle of the triangle. 385 views 2 years ago relationships within triangles. Web this page shows how to construct (draw) the incenter of a triangle with compass and straightedge or ruler. These midpoints will lie on the bisectors of the respective sides. Web to construct the incenter, first construct the three angle bisectors; To locate the incenter, one can draw each of the three angle bisectors, and then determine the point at which they all intersect. In this construction, we only use two, as this is sufficient to define the point where they intersect. Draw the perpendicular bisectors for the remaining sides of the triangle. 164k views 12 years ago special properties and parts of triangles | geometry | khan academy. Web © 2024 google llc. Take any two sides of the triangle and find their midpoints. The point where these three perpendicular bisectors intersect is the incenter of the triangle. You can also drag the origin point at (0,0). I = \left (\dfrac {15 \cdot 0+13 \cdot 14+14 \cdot 5} {13+14+15}, \dfrac {15 \cdot 0+13 \cdot 0+14 \cdot 12} {13+14+15}\right)=\left (6, 4\right).\ _\square i = ( 13+ 14+1515 ⋅0+13⋅14 +14⋅5, 13+ 14+1515⋅ 0+13⋅0+ 14⋅12) = (6,4). Construct a perpendicular from the incenter to one side of the triangle to locate the exact radius. Where all three lines intersect is the center of a triangle's incircle, called the incenter: Scroll down the page for more examples and solutions on the incenters of triangles. However, in coordinate geometry, we can use the formula to get the incenter. Web this page shows how to construct (draw) the incenter of a triangle with compass and straightedge or ruler. As can be seen in incenter of a triangle, the three angle bisectors of any triangle always pass through its incenter.
how to draw a incenter YouTube
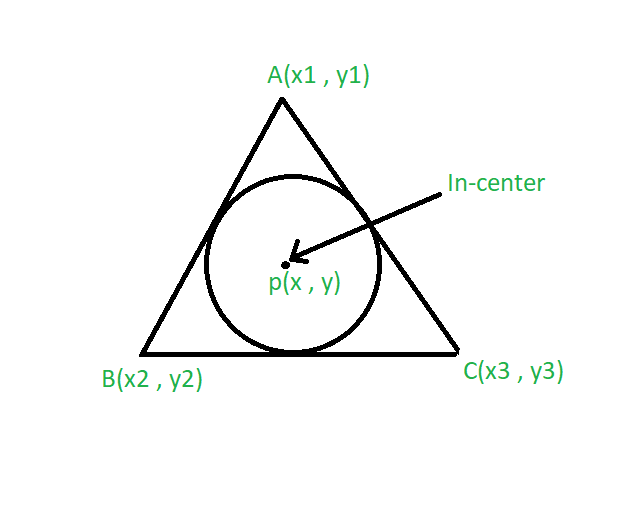
How To Find Incenter Of A Triangle With Coordinates We start with the

How to draw the Incenter and the Inscribed Circle of a triangle YouTube
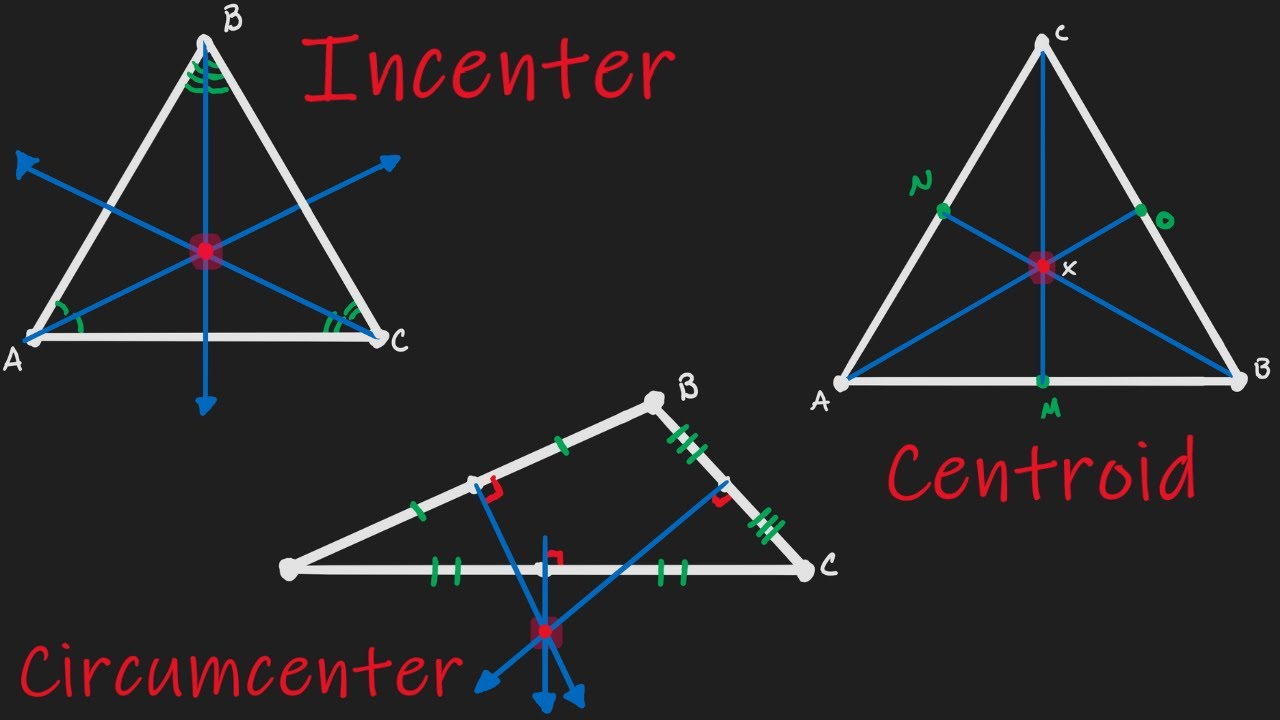
Incenter, Circumcenter and Centroid How to easily find these points

Incenter of a triangle Definition, Properties and Examples Cuemath

How to draw an incenter on geogebra YouTube

Incenter, Circumcenter, Orthocenter & Centroid of a Triangle Geometry

Incenter of a triangle Definition, Properties and Examples Cuemath

Incenter of a triangle Definition, Properties and Examples Cuemath
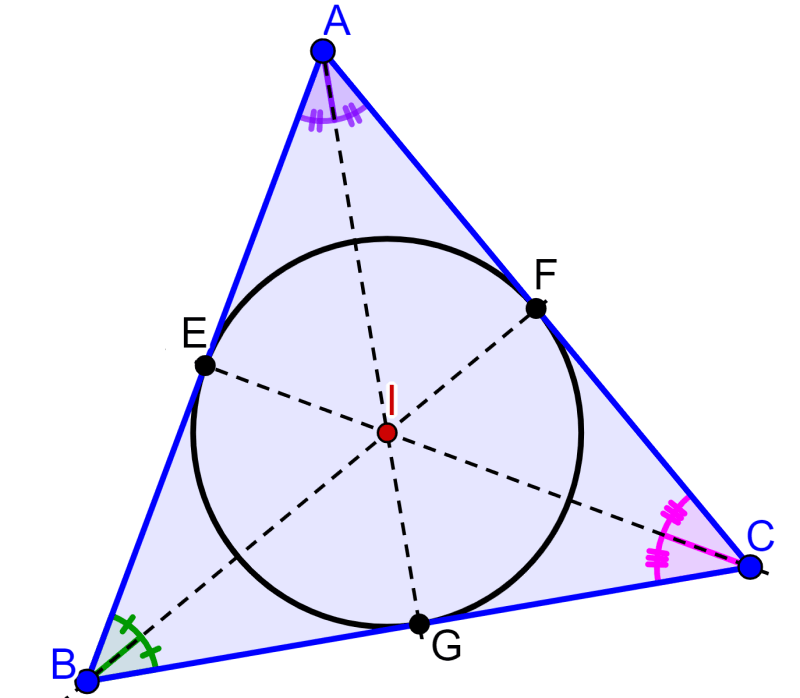
How to find the incenter of a triangle? Step by step Neurochispas
With Three Angle Bisectors, You Construct An Incenter.
The Incenter Is Always Located Within The Triangle.
Web Incenter (I) Of A Triangle = (Ax 1 + Bx 2 + Cx 3 /A + B + C, Ay 1 + By 2 + Cy 3 /A + B + C), Here Vertices A = (0, 0), B = (14, 0), And C = (5, 12)And Side Lengths Bc = 15, Ac = 13, & Ab = 14 I = (15 × 0 + 13 × 14 + 14 × 5/13 + 14 +.
The Incenter Of A Triangle Is The Point Where All Three Angle Bisectors Always Intersect, And Is The Center Of The Triangle's.
Related Post: