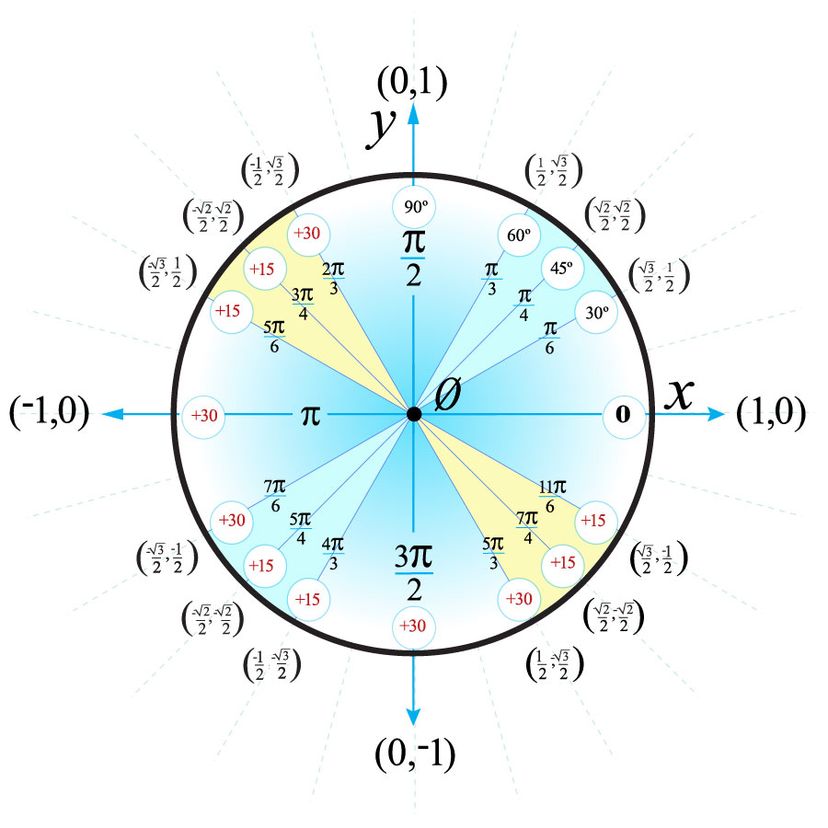
Unit Circle Drawing
Unit Circle Drawing - Cos (45 ° ) = √2 2. Web explore math with our beautiful, free online graphing calculator. The angle (in radians) that t intercepts forms an arc of length s. Web using the unit circle diagram, draw a line “tangent” to the unit circle where the hypotenuse contacts the unit circle. Sine, cosine and tangent (often shortened to sin, cos and tan) are each a ratio of sides of a right angled triangle: 1 2 , √2 2 and √3 2. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Web defining sine and cosine functions from the unit circle. In figure 2, the sine is equal to. Point (cosine of theta, sine of theta) is located near one thirty o'clock on the circle. Web to define our trigonometric functions, we begin by drawing a unit circle, a circle centered at the origin with radius 1, as shown in figure 2. Using the formula s = rt, and knowing that r = 1, we see that for a unit circle, s = t. 1 2 , √2 2 and √3 2. In fact, knowing. The angle (in radians) that t t intercepts forms an arc of length s. This line is at right angles to the hypotenuse at the unit circle and touches the unit circle only at that point (the tangent point). Cos (60 ° ) = √1 2 = 1 2. The angle (in radians) that t intercepts forms an arc of. No matter how big or small the triangle is. Web sine, cosine and tangent. In figure 2, the sine is equal to. In fact, knowing 3 numbers is enough: Web a unit circle is a circle with a radius measuring 1 unit. The angle (in radians) that t intercepts forms an arc of length s. Using the formula s = r t, s = r t, and knowing that r = 1, r = 1, we see that for a unit circle, s = t. Web a unit circle on an x y coordinate plane where the center of the unit circle. For a given angle θ each ratio stays the same. To help you remember, cos goes 3,2,1 cos (30 ° ) = √3 2. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Web to define our trigonometric functions, we begin by drawing a unit circle, a circle centered at the origin with radius 1, as. Cos (45 ° ) = √2 2. Web finding the function values for the sine and cosine begins with drawing a unit circle, which is centered at the origin and has a radius of 1 unit. In figure 2, the sine is equal to. Sine, cosine and tangent (often shortened to sin, cos and tan) are each a ratio of. Cos (60 ° ) = √1 2 = 1 2. Web a unit circle on an x y coordinate plane where the center of the unit circle is at the origin and the circumference of the circle touches (one, zero), (zero, one), (negative one, zero), and (zero, negative one). Cos (45 ° ) = √2 2. Web a unit circle. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Web sine, cosine and tangent. For a given angle θ each ratio stays the same. Web to define our trigonometric functions, we begin by drawing a unit circle, a circle centered at the origin with radius 1, as shown in figure 2. Using the formula s =. And, sin goes 1,2,3 : In a circle or on a graph. No matter how big or small the triangle is. Web a unit circle on an x y coordinate plane where the center of the unit circle is at the origin and the circumference of the circle touches (one, zero), (zero, one), (negative one, zero), and (zero, negative one).. In a circle or on a graph. The angle (in radians) that t intercepts forms an arc of length s. Graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. Sine, cosine and tangent (often shortened to sin, cos and tan) are each a ratio of sides of a right angled triangle: Web sine, cosine and tangent. In a circle or on a graph. Cos (45 ° ) = √2 2. Web a unit circle on an x y coordinate plane where the center of the unit circle is at the origin and the circumference of the circle touches (one, zero), (zero, one), (negative one, zero), and (zero, negative one). The angle (in radians) that t intercepts forms an arc of length s. Sine, cosine and tangent (often shortened to sin, cos and tan) are each a ratio of sides of a right angled triangle: Using the formula s = r t, s = r t, and knowing that r = 1, r = 1, we see that for a unit circle, s = t. In figure 2, the sine is equal to. This line is at right angles to the hypotenuse at the unit circle and touches the unit circle only at that point (the tangent point). Web explore math with our beautiful, free online graphing calculator. And, sin goes 1,2,3 : No matter how big or small the triangle is. The unit circle is generally represented in the cartesian coordinate plane. Sin (60 ° ) = √3 2. In fact, knowing 3 numbers is enough: 1 2 , √2 2 and √3 2. Web to define our trigonometric functions, we begin by drawing a unit circle, a circle centered at the origin with radius 1, as shown in figure 5.3.2.
Unit Circle Diagrams Classful
How to Use the Unit Circle in Trigonometry HowStuffWorks
Unit Circle Labeled With Special Angles And Values ClipArt ETC
Unit Circle Labeled With Quadrantal Angles And Values ClipArt ETC

Unit Circle Project Ideas Examples and Forms

Unit Circle Quick Lesson Downloadable PDF Chart · Matter of Math
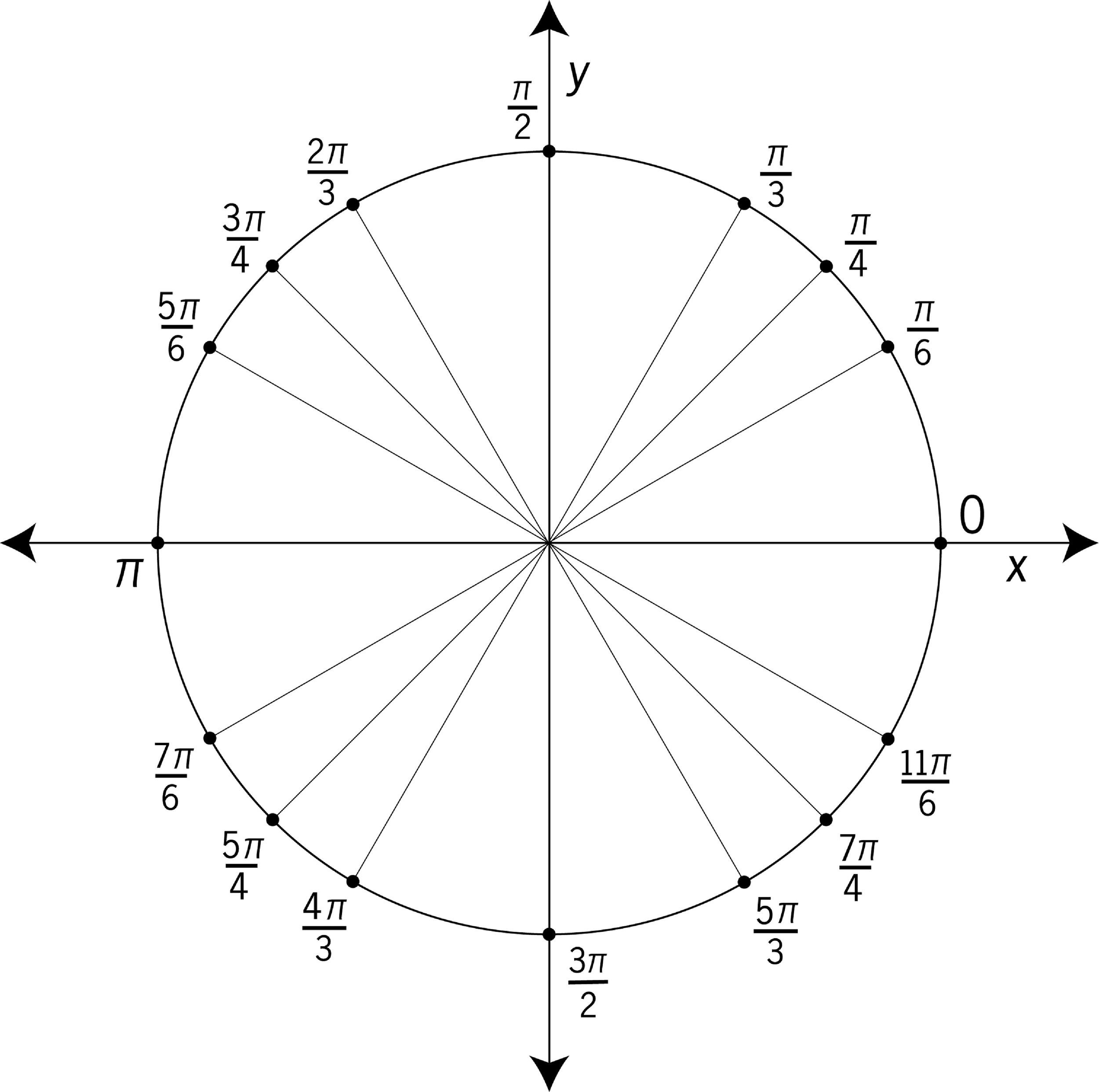
42 Printable Unit Circle Charts & Diagrams (Sin, Cos, Tan, Cot etc)

Unit Circle Quick Lesson Downloadable PDF Chart · Matter of Math

"How to draw an Unit Circle" UTRGV.241220C(Precalculus) YouTube
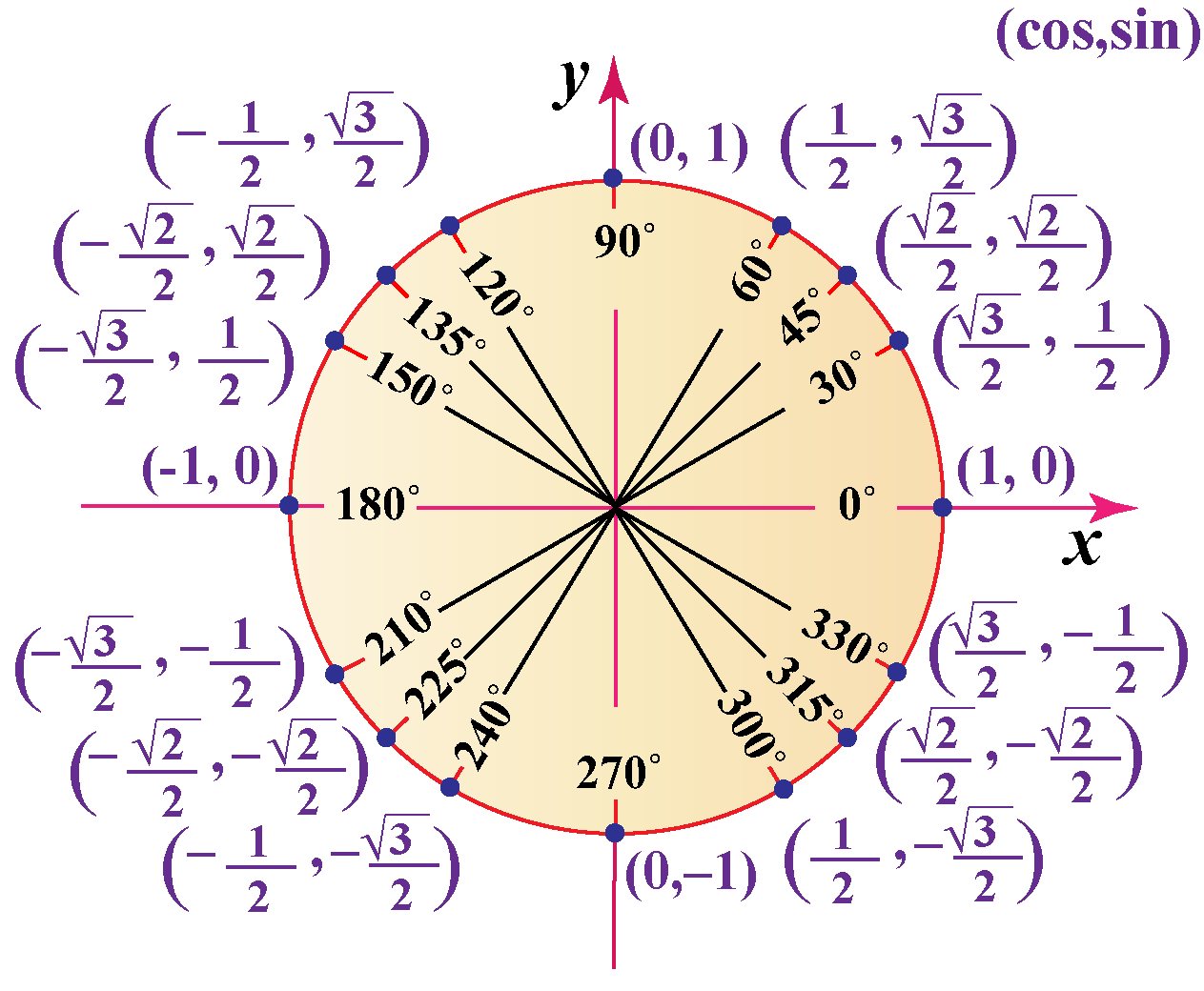
Unit circle Solved Examples Geometry Cuemath
Web Finding The Function Values For The Sine And Cosine Begins With Drawing A Unit Circle, Which Is Centered At The Origin And Has A Radius Of 1 Unit.
Sin (30 ° ) = √1 2 = 1 2 (Because √1 = 1) Sin (45 ° ) = √2 2.
The Angle (In Radians) That T T Intercepts Forms An Arc Of Length S.
Web Sine, Cosine And Tangent.
Related Post: